The photon mathematically
When you are interested in physics you must read “Unbelievable“!
The electron at rest has energy equal to:
![]()
The first part of the formula describes the electrostatic energy and the second part the magnetic spin energy. When the electron moves with speed v the interaction with the ether declines with the Lorentz-factor (Rv/Re). The charge of the electron is contracted to a smaller sphere. The radius Re shrinks to Rv and the potential energy increases therefore also with the Lorentz-factor:
![]()
The radius of the moving electron will be:
![]()
When the electron is halted the magnetic energy and the potential electrostatic energy are not in balance. The spin-energy of the electron (Re) is not sufficient anymore to confine the charge of the electron to a sphere with radius Rv. The electron will expand to Re, where the expanding potential force is again in equilibrium with contracting magnetic force of the spin energy. The overflow of magnetic- and kinetic energy of the electron will cause the electron to oscillate. The equation for the total energy of the electron can now be expressed as follows:
![]()
The third part of the equation, the oscillation energy hv, is the surplus energy of the electron. The oscillation energy expressed in the energy of the electron at rest is:
![]()
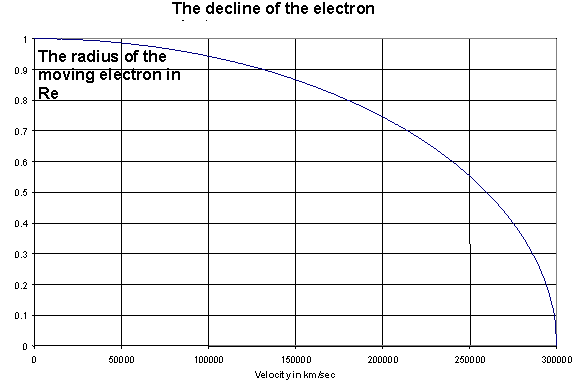
Figure 19. The declining radius of an electron in motion.
The photon oscillation frequency can be expressed in the “standard oscillation frequency” of the electron. The standard oscillation frequency of the electron ξ is the oscillation frequency of the electron when the oscillation energy is equal to the intrinsic energy of the electron at rest:
![]()
![]()
The frequency of the emitted photon can directly be calculated out of the speed v and standard oscillation frequency of the electron:
![]()
Next chapter: Time dilatation





